
Simplifying trigonometric expressions can be helpful when we are solving trigonometric equations or proving trigonometric identities. We can use the basic trigonometric ratios, combined and double-angle formulas, as well as reciprocal and other identities to do so.
The following are common formulas and identities we can use as tools to simplify trigonometric expressions:
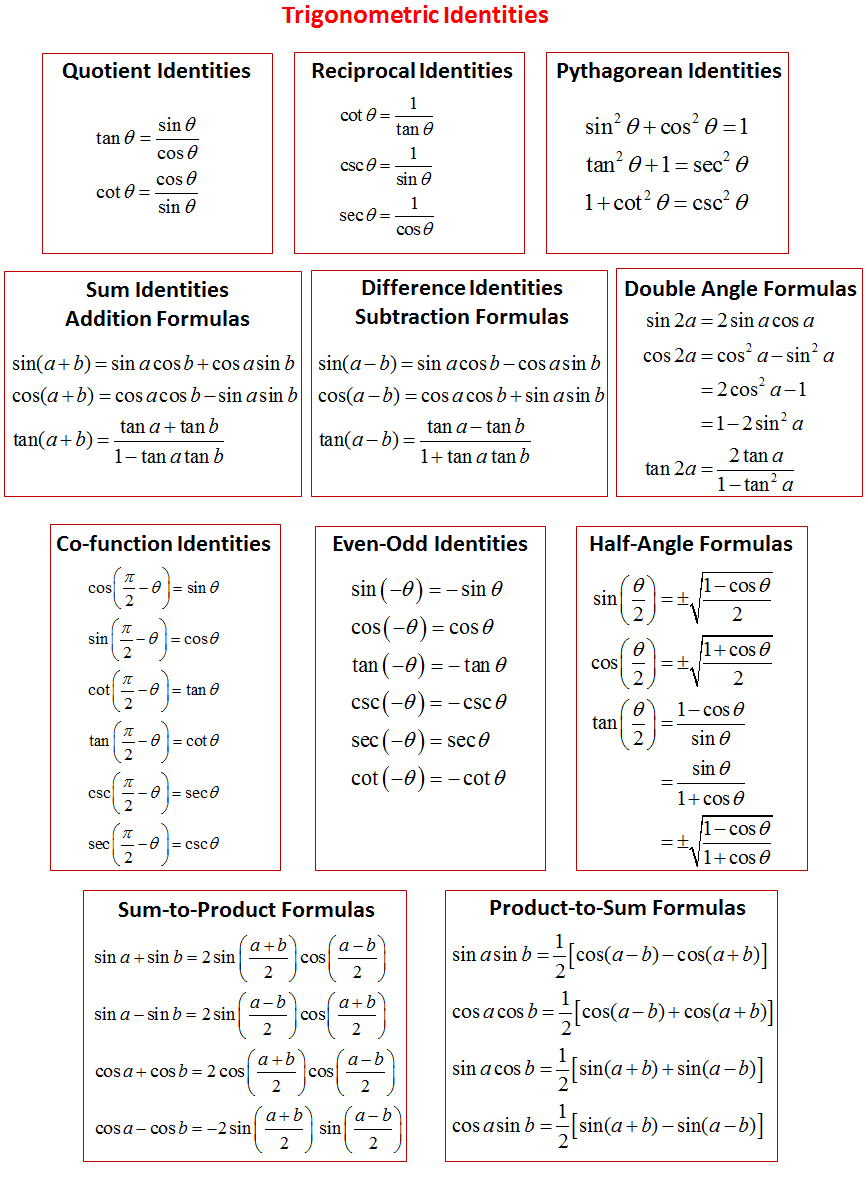
(Source: https://www.onlinemathlearning.com/image-files/trigonometric-identities.png)
Watch this video for proofs of the Pythagorean Identities:
You can use the information on the following image to remember the combined angle formulas:
See this video where we review the basic trigonometric ratios and identities that we can use to simplify trigonometric expressions, as well as solutions to the following example:
| 1. \((sin\theta\cdot tan\theta)(csc\theta+cot\theta)\) (starts at 1:59) | 2. \(\frac{tan(x)\cdot csc^2(x)}{1+tan^2(x)}\) (starts at 3:35) |
See this video where we review the combined angle formulas that we can use to simplify trigonometric expressions, as well as solutions to the following example:
| 1. \(sin3x\cdot cosx-sinx\cdot cos3x\) (starts at 5:09) | 2.\(cos(x+\pi)\cdot cos(x-\pi)+sin(x+\pi)\cdot sin(x-\pi)\) (starts at 6:03) |